Triangle center facts for kids

A triangle center is a special point inside or near a triangle. Think of it as a way to find the "middle" of a triangle. There are many different ways to find this center. Each method gives a different point and has its own name.

A triangle (ΔABC) with centroid (G), incenter (I), circumcenter (O), orthocenter (H) and nine-point center (N)

If a triangle has all sides equal (an equilateral triangle), all its centers are in the same spot. But for other triangles, these centers are in different places. Some centers can even be outside the triangle!

Ancient Greek mathematicians first found four important centers: the centroid, circumcenter, incenter, and orthocenter. Today, people have found over 40,000 triangle centers! You can find a list of them in the Encyclopedia of Triangle Centers.

What Makes a Point a Triangle Center?

A triangle center is a special kind of function that uses the three corners (called vertices) of a triangle. Every triangle center has two main rules it must follow.

Rule 1: Homogeneity

This rule means that if you change the size or position of a triangle, its center moves in the same way. For example, if you make a triangle twice as big, its center will also move to a spot that is twice as far from the original center. This applies to moving the triangle (translation), flipping it (reflection), turning it (rotation), or making it bigger or smaller (dilation).

Rule 2: Bisymmetry

This rule means that the order of the triangle's corners does not matter. If you have a triangle with corners A, B, and C, the center will be the same whether you list the corners as A, B, C or A, C, B. The center point does not change based on how you name the corners.

Important Triangle Centers

Many different points can be called triangle centers. Here are some of the most famous ones:

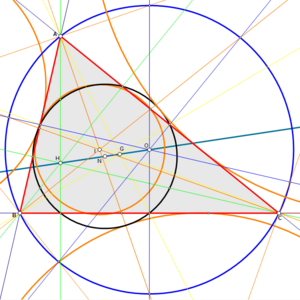
• Centroid - This is the point where the three medians of a triangle meet. A median is a line drawn from one corner of the triangle to the middle of the side opposite that corner. The centroid is like the triangle's balancing point.

• Circumcenter - This point is where the three perpendicular bisectors of the triangle's sides meet. A perpendicular bisector is a line that cuts a side exactly in half and forms a right angle with that side. The circumcenter is also the center of a circle that passes through all three corners of the triangle.

• Incenter - This is the point where the three angle bisectors of the triangle meet. An angle bisector is a line that cuts an angle of the triangle into two equal parts. The incenter is also the center of a circle that touches all three sides of the triangle.

• Orthocenter - This point is where the three altitudes of the triangle meet. An altitude is a line drawn from one corner of the triangle straight down to the opposite side, forming a right angle.

• Nine-point center - This is the center of a special circle called the nine-point circle. This circle passes through nine important points related to the triangle. These points include the middle of each side, the points where the altitudes touch the sides, and the middle points between the orthocenter and each corner.

• Fermat point - This is a special point inside a triangle where the total distance from this point to all three corners is as small as possible.

If a triangle is not an equilateral triangle, then the centroid, orthocenter, circumcenter, and nine-point center all lie on a single straight line. This line is called the Euler line, named after the famous mathematician Leonhard Euler.

See also

In Spanish: Elementos notables de un triángulo para niños