Mitchell Feigenbaum facts for kids
Quick facts for kids
Mitchell Feigenbaum
|
|
|---|---|

Mitchell Feigenbaum in 2006
|
|
| Born |
Mitchell Jay Feigenbaum
December 19, 1944 Philadelphia, Pennsylvania, US
|
| Died | June 30, 2019 (aged 74) New York City, New York, US
|
| Nationality | American |
| Alma mater | City College of New York (BS) Massachusetts Institute of Technology (PhD) |
| Known for | Feigenbaum constants Feigenbaum function Feigenbaum universality |
| Awards | MacArthur Fellow (1984) Wolf Prize (1986) Heineman Prize (2008) |
| Scientific career | |
| Fields | Mathematical physics |
| Institutions | Rockefeller University |
| Doctoral advisor | Francis E. Low |
Mitchell Jay Feigenbaum (born December 19, 1944 – died June 30, 2019) was an American scientist. He was a mathematical physicist, which means he used math to study physics. His important work helped create a field called chaos theory. He is famous for discovering the Feigenbaum constants. These are special numbers that help us understand how complex systems behave.
Contents
Early Life and Education
Mitchell Feigenbaum was born in Philadelphia, Pennsylvania. His parents had moved to the United States from Poland and Ukraine. He went to Samuel J. Tilden High School in Brooklyn, New York. Later, he studied at the City College of New York.
In 1964, he started his advanced studies at the Massachusetts Institute of Technology (MIT). He first planned to study electrical engineering. But he soon changed his focus to physics. He earned his PhD in 1970. His main teacher for his doctorate was Professor Francis E. Low.
Career and Discoveries
After finishing his studies, Feigenbaum worked at a few universities. These included Cornell University and the Virginia Polytechnic Institute and State University. In 1974, he got a job at the Los Alamos National Laboratory in New Mexico. There, he studied how liquids move in a messy way, which is called turbulence.
Even though a full theory of turbulence is still being developed, his research led him to study something else. He started looking at chaotic maps. These are mathematical rules that can show very complex and unpredictable patterns.
In 1983, he received a special award called the MacArthur Fellowship. This award is given to talented people to support their creative work. In 1986, he won the Wolf Prize in Physics. He shared this award with Albert Libchaber. They were honored for showing how many different systems in nature behave in a similar way. This helped scientists to study chaos in a more organized way.
Feigenbaum worked as a professor at Rockefeller University from 1986 until he passed away.
Understanding Chaos and the Feigenbaum Constants
Mitchell Feigenbaum's most famous work is about how simple mathematical rules can lead to complex, seemingly random behavior. This is known as chaos theory. He studied mathematical mappings, which are like formulas that take a number and give you a new one.
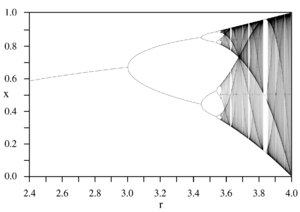
He noticed that as you change a certain value in these formulas, the behavior changes. First, you might get one steady answer. Then, it might split into two different answers that it switches between. Then it splits again into four answers, and so on. This splitting is called a bifurcation.
In 1975, Feigenbaum made an amazing discovery using a small calculator. He found that the ratio of the distances between these splitting points was always the same. This ratio was about 4.6692... This number is now known as the first Feigenbaum constant.
He showed that this same constant appears in many different types of mathematical functions. This was a huge step for scientists. It meant they could start to understand the "random" behavior of chaotic systems. His work helped explain how complex patterns can come from simple rules.
One important example he studied was the logistic map. This is a simple formula that can show very complex behavior. His findings were published in a famous article in 1978.
Other Contributions
Feigenbaum also helped develop new ways to create fractal maps. Fractals are complex shapes that look the same no matter how much you zoom in. He worked with a company called Hammond to use computers to draw maps better.
In 1996, he started a company called Numerix with Michael Goodkin. This company created software that helped financial companies. It made it much faster to calculate the value of complex financial products. Numerix is still a leading company in this field today.
Works
- Feigenbaum, Mitchell J. (May 1983). "Universal behavior in nonlinear systems". Physica D: Nonlinear Phenomena 7 (1-3): 16–39. doi:10.1016/0167-2789(83)90112-4. http://cs.physics.sunysb.edu:80/verbaarschot/html/lectures/phy501-07/chaos/getfile.pdf. "A semipopular account of the universal scaling theory for the period doubling route to chaos is presented.".
- Feigenbaum, Mitchell J. (1 July 1978). "Quantitative universality for a class of nonlinear transformations". Journal of Statistical Physics 19 (1): 25–52. doi:10.1007/BF01020332.
- Feigenbaum, Mitchell J. (March 1987). "Some characterizations of strange sets". Journal of Statistical Physics 46 (5-6): 919–924. doi:10.1007/BF01011148.
See also
 In Spanish: Mitchell Feigenbaum para niños
In Spanish: Mitchell Feigenbaum para niños