First order logic facts for kids
First-order logic is a special kind of logic used in mathematics and philosophy. Think of it as a powerful language that helps us describe and reason about things in a very precise way. It lets us build rules and statements without needing to know exactly what those statements are about at first.
This type of logic helps us make arguments and draw conclusions. It's different from a simpler type called propositional logic because first-order logic has special words called quantifiers. These quantifiers let us talk about "all" things or "at least one" thing.
For example:
- For all (written as
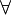): This means "for every single one" or "for all of them." - There is at least one (written as
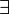): This means "there is one or more" or "some exist."
First-order logic also uses ideas like negation (saying "not"), conjunction (saying "and"), inclusive disjunction (saying "or"), exclusive disjunction (saying "either... or, but not both"), and implication (saying "if... then..."). These are the same as in propositional logic. Because it adds quantifiers, first-order logic is like an upgraded version of propositional logic.
Why is First-Order Logic Important?
A famous mathematician named Kurt Gödel showed something very important about first-order logic. He proved that if a statement is true in first-order logic, you can always prove it using the rules of the logic. This is called the "completeness" of first-order logic.
First-order logic, along with Zermelo–Fraenkel set theory (a way to think about collections of things), forms the basic building blocks for many parts of modern mathematics. It helps mathematicians make sure their ideas and proofs are solid and correct.
Related pages
See also
In Spanish: Lógica de primer orden para niños
