Cellular automaton facts for kids
A cellular automaton is like a special grid or board game. It's a model used in computer science and mathematics. Imagine a grid made of many small squares, called "cells." Each cell can be in one of a few different states, like "on" or "off," or a certain color.
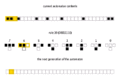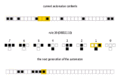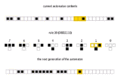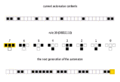
What makes it special is how the cells change. In each "turn" or step, a cell's state changes based on two things: what state it's currently in, and what states its nearby cells (its neighbors) are in. This creates interesting patterns and behaviors over time. A very famous example is Conway's Game of Life, which was first shown in the 1970s. Scientists Stanislaw Ulam and John von Neumann first talked about cellular automata in the 1940s.
Contents
Cellular Automata in Nature
Did you know that some things in nature work like cellular automata? Scientists can also use these models to understand how certain biological processes happen.
Seashell Patterns
The beautiful patterns you see on some seashells are made by natural cellular automata. For example, shells from the Conus and Cymbiola groups show this. These shells have special cells along their edge that make pigment (color). Each cell releases color based on what its neighbor cells are doing. As the shell slowly grows, this band of cells leaves behind the colored pattern. The Conus textile shell, for instance, has a pattern that looks very much like a famous cellular automaton rule called rule 30.
Plant Breathing
Plants also use a cellular automaton-like system to control how they take in and let out gases. Tiny openings on a leaf are called stomata. Each stoma acts like a cell in a cellular automaton, helping the plant "breathe."
Animal Skin Patterns
The changing wave patterns on the skin of cephalopods (like octopuses and squids) can be copied using a simple cellular automaton model. Each cell in the model can be either "expanded" or "retracted," just like the color cells (chromatophores) on the animal's skin.
Brain Cells and Learning
Scientists have even created cellular automata models to act like neurons, which are brain cells. These models can help us understand how complex things like recognizing objects or learning new things might happen in the brain.
Fibroblast Cells
Fibroblasts are a type of cell in our bodies. They act a bit like cellular automata because each fibroblast mostly interacts with the cells right next to it.
Related Pages
Images for kids
-
Gosper's Glider Gun creating "gliders" in the cellular automaton Conway's Game of Life
-
A torus, a donut-shaped object
-
John von Neumann, from his ID badge at Los Alamos
See also
 In Spanish: Autómata celular para niños
In Spanish: Autómata celular para niños