Chaos theory facts for kids
Chaos theory is a part of mathematics. It looks at certain systems that are very sensitive. A very small change may make the system behave completely differently.
Very small changes in the starting position of a chaotic system make a big difference after a while. This is why even large computers cannot tell the weather for more than a few days in the future. Even if the weather was perfectly measured, a small change or error will make the prediction completely wrong. Since even a butterfly can make enough wind to change weather, a chaotic system is sometimes called the "butterfly effect". No computer knows enough to tell how the small wind will change the weather.
Some systems (like weather) might appear random at first look, but chaos theory says that these kinds of systems or patterns may not be. If people pay close enough attention to what is really going on, they might notice the chaotic patterns.
The main idea of chaos theory is that a minor difference at the start of a process can make a major change in it as time progresses. Quantum chaos theory is a new idea in the study of chaos theory. It deals with quantum physics.
Contents
Examples
As an example, take a pendulum that is attached at some point, and swings freely. Connecting a second pendulum to the first will make the system completely different. It is very hard to start in exactly the same position again - a change in starting position so small that it cannot even be seen can quickly cause the pendulum swing to become different from what it was before.
A very important part to the study of chaos theory is the study of mathematics functions that are known as fractals. Fractal functions work like chaotic systems: a small change in the starting values can change the value of the function in ways that look random. Due to the fact that they are mathematical, they are easy to study.
Related pages
Images for kids
-
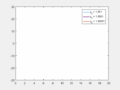
Lorenz equations used to generate plots for the y variable. The initial conditions for x and z were kept the same but those for y were changed between 1.001, 1.0001 and 1.00001. The values for \rho, \sigma and \beta were 45.92, 16 and 4 respectively. As can be seen from the graph, even the slightest difference in initial values causes significant changes after about 12 seconds of evolution in the three cases. This is an example of sensitive dependence on initial conditions.
-
Six iterations of a set of states [x,y] passed through the logistic map. The first iterate (blue) is the initial condition, which essentially forms a circle. Animation shows the first to the sixth iteration of the circular initial conditions. It can be seen that mixing occurs as we progress in iterations. The sixth iteration shows that the points are almost completely scattered in the phase space. Had we progressed further in iterations, the mixing would have been homogeneous and irreversible. The logistic map has equation x_{k+1} = 4 x_k (1 - x_k ). To expand the state-space of the logistic map into two dimensions, a second state, y, was created as y_{k+1} = x_k + y_k , if x_k + y_k
-
The map defined by x → 4 x (1 – x) and y → (x + y) mod 1 also displays topological mixing. Here, the blue region is transformed by the dynamics first to the purple region, then to the pink and red regions, and eventually to a cloud of vertical lines scattered across the space.
-
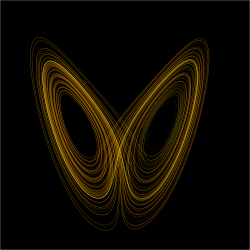
The Lorenz attractor displays chaotic behavior. These two plots demonstrate sensitive dependence on initial conditions within the region of phase space occupied by the attractor.
-
frameless
-
Barnsley fern created using the chaos game. Natural forms (ferns, clouds, mountains, etc.) may be recreated through an iterated function system (IFS).
-
Turbulence in the tip vortex from an airplane wing. Studies of the critical point beyond which a system creates turbulence were important for chaos theory, analyzed for example by the Soviet physicist Lev Landau, who developed the Landau-Hopf theory of turbulence. David Ruelle and Floris Takens later predicted, against Landau, that fluid turbulence could develop through a strange attractor, a main concept of chaos theory.
-
A conus textile shell, similar in appearance to Rule 30, a cellular automaton with chaotic behaviour.
-
The red cars and blue cars take turns to move; the red ones only move upwards, and the blue ones move rightwards. Every time, all the cars of the same colour try to move one step if there is no car in front of it. Here, the model has self-organized in a somewhat geometric pattern where there are some traffic jams and some areas where cars can move at top speed.
See also
In Spanish: Teoría del caos para niños
