Electrical conductivity facts for kids
Electrical conductivity measures how easily electricity can pass through a material. Think of it as how "slippery" a material is for electric charges. The better a material conducts, the higher its conductivity.
The special unit for electrical conductivity is the siemens per metre, often written as Sm−1. This unit is named after Werner von Siemens.
Electrical conductivity is the opposite of electrical resistivity, which measures how much a material resists the flow of electricity.
Materials like metals are good conductors because they have high conductivity. Insulators like glass or plastic have very low conductivity. Then there are semiconductors, which can change how well they conduct electricity depending on the conditions.
Contents
Why Materials Conduct Electricity
Electricity flows when tiny charged particles, usually electrons, can move freely through a material. When you apply an electric push (like from a battery) across a conductor, these electrons start to move, creating an electric current.
How Electrons Move in Materials
Inside atoms and materials, electrons can only exist at certain energy levels. Imagine these levels as steps on a ladder. When many steps are very close together, they form an "energy band." Materials have different energy bands.
Electrons naturally try to settle into the lowest possible energy steps. However, a rule called the Pauli exclusion principle means that no two electrons can be in exactly the same state. So, electrons fill up the energy bands from the bottom.
The "Fermi level" is like the highest step that electrons have filled up. Only electrons near this Fermi level can easily move around and conduct electricity. Electrons in lower, completely filled steps are stuck, and higher, empty steps have no electrons to move.
Conductivity in Metals
Metals are made of a structure of atoms. Each atom has outer electrons that can break free and travel through the metal's structure. This creates a "sea" of free electrons. This is why metals are such good conductors.
When you connect a metal wire to a voltage source, the free electrons start to drift towards the positive end. Even though the electrons themselves move quite slowly (only about a meter per hour!), the electrical signal travels through the metal almost at the speed of light. It's like how energy moves through a Newton's cradle – the balls themselves don't travel far, but the push moves quickly.
Most metals have some resistance. This happens because as electrons move, they bump into the atoms in the metal's structure. The more regular the structure, the less resistance there is.
Two main things affect a metal's resistance:
- Temperature: When a metal gets hotter, its atoms vibrate more. This makes it harder for electrons to pass through, increasing resistance.
- Impurities: If a metal isn't pure and has other types of atoms mixed in, these impurities create bumps and irregularities that increase resistance.
Also, a thicker wire has more space for electrons to flow, so it has lower resistance. A longer wire means electrons have to travel further and hit more obstacles, so it has higher resistance.
Conductivity in Semiconductors and Insulators
In semiconductors, the electrons are not as free as in metals. At very cold temperatures, semiconductors act like insulators and don't conduct electricity well. However, as they get warmer, or if special "dopant" atoms are added, more electrons become free to move. This makes semiconductors useful for electronics, as their conductivity can be controlled.
Insulators have very few free electrons, so electricity cannot easily flow through them.
Conductivity in Liquids (Electrolytes)
In some liquids, like salt water, electricity is carried by charged atoms or molecules called ions, not by electrons. These ions move through the liquid, carrying their electric charge. Pure water is a poor conductor, but adding salt makes it a good conductor because of the ions.
Inside living things, like in our bodies, electrical signals are carried by ions moving through special channels in cell membranes.
Superconductivity
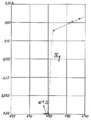
Normally, as you cool a metal, its electrical resistance goes down. But even at extremely cold temperatures, regular metals still have some resistance.
However, in a superconductor, something amazing happens: when it's cooled below a certain "critical temperature," its electrical resistance completely disappears! This means electricity can flow through a loop of superconducting wire forever without any power source.
In 1986, scientists found new types of ceramic materials that become superconductors at much warmer (though still very cold) temperatures. These are called high-temperature superconductors. Some can even work when cooled with Liquid nitrogen, which is much easier to use than other super-cold methods.
Plasma Conductivity

Plasma is often called the "fourth state of matter" (after solid, liquid, and gas). It's a gas where many of the atoms have lost or gained electrons, becoming ions. This means plasma is full of free electrons and ions.
Because it has so many charged particles, plasma is a very good electrical conductor. Electric fields play a big role in how plasma behaves. For example, Lightning is a natural example of plasma. It can carry huge electric currents and reach incredibly high temperatures.
Scientists study how plasmas interact with magnetic fields in a field called magnetohydrodynamics. This helps us understand things like the Sun, stars, and fusion energy.
Images for kids
-
Original data from the 1911 experiment by Heike Kamerlingh Onnes showing the resistance of a mercury wire as a function of temperature. The abrupt drop in resistance is the superconducting transition.
See also
 In Spanish: Conductividad eléctrica para niños
In Spanish: Conductividad eléctrica para niños