Symmetry facts for kids
Symmetry is about things looking balanced and beautiful. Think of a butterfly: its left wing looks just like its right wing. That's symmetry!
In mathematics, symmetry means something stays the same even after you move it or change it in a certain way. This could be by sliding it, flipping it, spinning it, or making it bigger or smaller.
Both everyday ideas of symmetry and the math definition are connected. This article will explore symmetry in math, science, nature, and art.
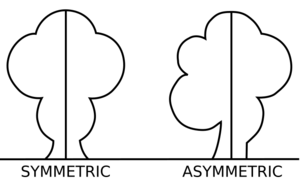
The opposite of symmetry is asymmetry. This means something does not have symmetry.
Contents
What is Symmetry?
Symmetry in Math and Shapes
When a shape or object is symmetric, it means you can divide it into two or more parts that are exactly alike. These parts are arranged in a neat way. An object is symmetric if you can move its parts without changing the whole shape. The type of symmetry depends on how the parts are arranged or how you transform the object.
Here are some types of symmetry:
- Reflectional Symmetry: This is also called mirror symmetry. If you can draw a line through an object and one side is a perfect mirror image of the other, it has reflectional symmetry. Think of folding a piece of paper in half.
- Rotational Symmetry: An object has this if you can spin it around a central point and it looks the same before it completes a full circle. A pinwheel has rotational symmetry.
- Translational Symmetry: This happens when you can slide an object (move every part the same distance) and it still looks the same. A repeating pattern on wallpaper often has translational symmetry.
- Helical Symmetry: This is found in 3D objects. It means you can slide and rotate an object at the same time along a line, and it looks the same. A spiral staircase is a good example.
- Scale Symmetry: An object has scale symmetry if it looks the same when you make it bigger or smaller. Fractals are shapes where smaller parts look like the whole shape.
Symmetry in Logic
In logic, symmetry can describe how ideas or statements relate to each other. For example, if "Paul is the same age as Mary" is true, then "Mary is the same age as Paul" must also be true. This relationship is symmetric.
Some logical words are symmetric, like "and" or "or." If "A and B" is true, then "B and A" is also true.
Symmetry in Other Math Areas
Beyond shapes, symmetry is a big idea in many parts of math. It means that an operation (like adding or multiplying) keeps some property of an object the same. The set of operations that do this often form a "group."
For example, in calculus, some functions are called "even" or "odd" based on their symmetry. In statistics, some data patterns are called "symmetric distributions" if they are balanced.
Symmetry in Science and Nature
Symmetry in Physics
In physics, symmetry means that something doesn't change even when you transform it. This idea is very powerful! Many laws of nature come from symmetries. For example, a rule called Noether's theorem says that for every continuous symmetry, there's something that stays constant, like energy or momentum.
Important symmetries in physics include how things behave in space and time, and how tiny particles are structured.
Symmetry in Biology
In biology, symmetry often describes the shapes of living things.
- Bilateral Symmetry: Many animals, including humans, have bilateral symmetry. This means they can be divided into two nearly identical left and right halves. Animals that move forward, like us, usually have a head, a tail, and a left and right side. This helps with movement. Even though our outside is symmetric, our inside organs often are not!
- Radial Symmetry: Plants and animals that don't move much, like sea anemones, often have radial symmetry. This means they look the same from many different angles around a central point. This is useful because food or danger can come from any direction.
- Five-fold Symmetry: You can see this in echinoderms, like starfish and sea urchins. They have five parts arranged around a center.
Symmetry also helps scientists understand how living things change over time through biological evolution.
Symmetry in Chemistry
Symmetry is very important in chemistry. It helps explain how molecules interact with each other. For example, many molecules in nature are "chiral," meaning they are like a left or right hand – they are mirror images but can't be perfectly placed on top of each other. This is key for how medicines work in our bodies.
Understanding the symmetry of molecules helps scientists create new drugs with fewer side effects. Symmetry also explains basic ideas in quantum chemistry and how we study materials using tools like spectroscopy and crystallography.
Images for kids
-
Leonardo da Vinci's Vitruvian Man (around 1487) shows symmetry in the human body.
-
Symmetric arches in the Great Mosque of Kairouan in Tunisia.
-
Clay pots made on a pottery wheel have rotational symmetry.
See also
 In Spanish: Simetría para niños
In Spanish: Simetría para niños
- Automorphism
- Chirality
- Even and odd functions
- Palindrome
- Spacetime symmetries
- Symmetric relation
- Symmetry group